Beer is greatest served chilly, and it seems that math may help with that. Cláudio de Castro Pellegrini of the Federal College of São João del-Rei in Brazil lately got down to formulate an equation to find out the optimum form of a beer glass that can hold a beverage tastily chilled—in different phrases, he was on the lookout for a glass that forestalls the liquid in it from absorbing warmth.
The only strategy can be to discover a vessel whose floor space is as small as potential relative to its quantity. That’s as a result of the warmth from the glass’s environment penetrates the floor of the glass. The smaller this floor is, the much less warmth will get in, and the longer the beer stays pleasantly cool. Way back to historical occasions, students acknowledged that within the case of two dimensions, a circle gives a minimal ratio of circumference to space. And this discovering additionally applies in three dimensions: a sphere has the smallest potential floor space in contrast with its quantity.
However a spherical beer glass can be unwieldy. What’s extra, Pellegrini was not desirous about a static scenario by which you allow beer in a glass and watch the liquid heat up. “The process is quite straightforward here: a request is made for a beer, the waiter delivers it, it is served, it is consumed. Repeat.” he wrote in a preprint paper describing the analysis, which was posted in October to the server arXiv.org. Because of this the extent contained in the glass modifications, and so does the floor that’s involved with the surroundings. (As extra of the glass is drained, extra of its floor touches the air round it.)
On supporting science journalism
Should you’re having fun with this text, take into account supporting our award-winning journalism by subscribing. By buying a subscription you’re serving to to make sure the way forward for impactful tales concerning the discoveries and concepts shaping our world in the present day.
Pellegrini began with some constraints on the form of the glass. It ought to encompass an insulating, flat base that’s giant sufficient to make the glass stand upright. As well as, the glass needs to be symmetrical in order that its form is represented by a physique of revolution, or a three-dimensional form created by rotating a two-dimensional one 360 levels across the x axis or y axis. To find out an optimum beer glass form, Pellegrini adjusted the 2D form that defines the physique of revolution. In an earlier preprint evaluation, detailed on arXiv.org in February, he described optimum glass shapes on this method, however probably the most possible vessels had a quantity of greater than two liters—and the biggest glass had a quantity of greater than 100 liters.
Subsequent, Pellegrini aimed to design a practical beer glass. However he needed to make some simplifications. He assumed that the bottom would insulate warmth completely. (In actuality, a thick glass base and using coasters assist, however excellent insulation is unattainable.) He additionally assumed that the temperature of the beer can be the identical in all places within the liquid and that the beverage would have a uniform density, which is usually true for filtered varieties. Pellegrini ignored the beer’s foam as a potential insulating layer. And eventually, he additionally ignored the warmth switch from an individual’s hand to the glass, solely investigating the results of the ambient temperature. “In the most critical scenario, such as at the beach on a [38-degree-Celsius] windy day, as few as 3 minutes may be sufficient (again based on personal experience, exhaustively repeated) to render the beer undrinkable,” Pellegrini wrote.
A Method for the Optimum Beer Glass
With these assumptions, the researcher created an equation that describes the change in temperature of the beer over time. This can be a differential equation, that means the method incorporates a by-product: a operate that exhibits the speed of change (of temperature, on this case) with respect to a variable, which on this scenario is the beer glass form:
Right here, T is the beer temperature, TU is the ambient temperature, cp is the particular warmth capability (how arduous it’s to warmth up a substance), ρ is the density, V is the amount, Atot is the uncovered floor of the beer (together with the aspect and the higher round floor) and hCV is the convective warmth switch coefficient, representing its capability to conduct warmth. Utilizing this method, Pellegrini calculated 4 ideas to make sure that beer cools down as slowly as potential.
At greatest, it is best to benefit from the beer in a cool place in order that TU is as little as potential.
Should you use a extra insulating materials comparable to ceramic as a substitute of glass, the warmth conduction coefficient decreases.
Typically, Pellegrini identified {that a} thick head of froth insulates the beer and protects it from shedding carbon dioxide too shortly. As well as, wind and drafts needs to be averted as a result of these result in compelled convection, which performs a a lot larger position in warmth switch than the pure convection thought-about right here.
All of the following pointers can often be adopted solely by transferring to a unique location. If you’re sitting comfortably in a bar, you’re uncovered to the native circumstances, nevertheless, so it will be all of the extra welcome if, from the outset, bars had been to make use of glasses whose form was optimized for minimal warmth alternate. Spoiler: this isn’t the case.
The Beer Champagne Flute
The form of the beer glass is contained within the quotient Atot⁄V within the above equation. The smaller that quantity, the smaller the temperature change. That’s why Pellegrini centered on minimizing the worth of Atot⁄V.
At college, mathematicians study that such optimization duties might be solved with derivatives. For instance, to search out the vertex of a parabola f(x) = x2 + 3x + 2, the operate is derived from x and the result’s set to zero: 0 = 2x + 3. Because of this the vertex might be discovered at x = –1.5.
Pellegrini proceeded in precisely the identical method: He derived the quotient Atot/V, set it to zero after which used the properties of our bodies of revolution to derive a operate for the optimum form of a beer glass. In response to this, the radius r of an ideal beer glass as a operate of the peak h is given by the next method:

C is a continuing, and Rb is the radius of the glass base. The method exhibits that the mouth opening of the glass needs to be giant, and the glass ought to grow to be narrower towards the underside, just like many frequent beer glasses. The radius of real-world glasses isn’t all the time progressively smaller, nevertheless—they typically have a curved S form, as seen from the aspect. This distinguishes them from an ideal beer glass.

The precise form of the optimum glass relies on the radius of the glass base and the fixed C, which is decided by the peak of the glass, amongst different issues. Pellegrini first calculated the optimum shapes for glasses with a top of 19 centimeters for various base radii. The ensuing form is paying homage to a champagne flute.
The engineer then tailored the parameters of the right glass to several types of beer glass, such because the Imperial pint, the American pint or a Weizen (wheat beer) glass, that are extensively used within the U.Ok. To do that, Pellegrini entered the respective base radius and top into his method to calculate the optimum form.
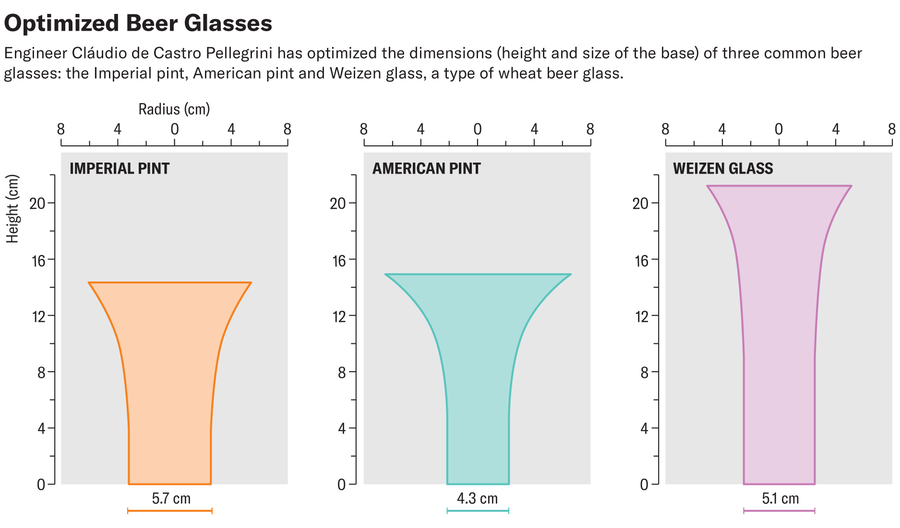
Right here, too, the tough form seems to be the identical for all sorts and is paying homage to a champagne flute. However even nonoptimal glasses have some advantages, Pellegrini wrote within the preprint. Though the Nadir Figueiredo glass, which is widespread in Brazil, are removed from the optimum form, they’re nonetheless completely designed for warmth transport in sure respects: as a result of they maintain a comparatively small quantity of beer, folks often drink the beer sooner than it may heat considerably.
This text initially appeared in Spektrum der Wissenschaft and was reproduced with permission.

