The quantity pi (π) seems in essentially the most unlikely locations. It may be present in circles, in fact—in addition to in pendulums, springs and river bends. This on a regular basis quantity is linked to transcendental mysteries. It has impressed Shakespearean thought puzzles, baking challenges and even an authentic track. And pi retains the surprises coming—most just lately in January 2024, when physicists Arnab Priya Saha and Aninda Sinha of the Indian Institute of Science offered a utterly new system for calculating it, which they later printed in Bodily Overview Letters.
Saha and Sinha aren’t mathematicians. They weren’t even on the lookout for a novel pi equation. Fairly, these two string theorists have been engaged on a unifying concept of elementary forces, one that might reconcile electromagnetism, gravity and the robust and weak nuclear forces. In string concept, the fundamental constructing blocks of the universe aren’t particles, comparable to electrons or photons, however quite tiny threads that vibrate just like the strings of a guitar and in so doing trigger all seen phenomena. Of their work, Saha and Sinha have investigated how these strings might work together with one another—and by accident found new formulation which are associated to essential mathematical portions.
For millennia, mankind has been attempting to find out the precise worth of pi. This isn’t shocking, given the utility of calculating the circumference or space of a circle, which pi allows. Even historic students developed geometric approaches to calculate this worth. One well-known instance is Archimedes, who estimated pi with the assistance of polygons: by drawing an n-sided polygon inside and one exterior a circle and calculating the perimeter of every, he was capable of slender down the worth of pi.
On supporting science journalism
If you happen to’re having fun with this text, contemplate supporting our award-winning journalism by subscribing. By buying a subscription you’re serving to to make sure the way forward for impactful tales in regards to the discoveries and concepts shaping our world at present.
A standard technique for figuring out pi geometrically includes drawing a bounding polygon inside and outdoors a circle after which evaluating the 2 perimeters.
Fredrik/Leszek Krupinski/Wikimedia Commons
Lecturers usually current this technique in class. However even in case you don’t bear in mind it, you possibly can most likely think about that the method is sort of advanced. Archimedes went as far as to check the sides of polygons with 96 vertices to show that pi is between 3.1408 and three.1429. This strategy is subsequently probably not sensible for calculating pi precisely.
An Infinite Sequence to Decide Pi
Within the fifteenth century consultants discovered infinite collection as a brand new strategy to categorical pi. By including up their numbers one after the other, pi’s worth may be obtained. And the extra summands you have a look at, the extra correct the end result turns into.
For instance, the Indian scholar Madhava, who lived from 1350 to 1425, discovered that pi equals 4 multiplied by a collection that begins with 1 after which alternately subtracts or provides fractions wherein 1 is positioned over successively increased odd numbers (so 1/3, 1/5, and so forth). One strategy to categorical this is able to be:

This system makes it potential to find out pi as exactly as you want in a quite simple manner. You don’t should be a grasp of arithmetic to work out the equation. However you do want endurance. It takes a very long time to get correct outcomes. Even in case you consider 100 summands, you’ll nonetheless be far off the mark.
As Saha and Sinha found greater than 600 years later, Madhava’s system is barely a particular case of a way more normal equation for calculating pi. Of their work, the string theorists found the next system:
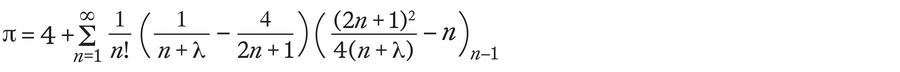
This system produces an infinitely lengthy sum. What’s hanging is that it depends upon the issue λ , a freely selectable parameter. It doesn’t matter what worth λ has, the system will all the time end in pi. And since there are infinitely many numbers that may correspond to λ, Saha and Sinha have discovered an infinite variety of pi formulation.
If λ is infinitely giant, the equation corresponds to Madhava’s system. That’s, as a result of λ solely ever seems within the denominator of fractions, the corresponding fractions for λ = ∞ develop into zero (as a result of fractions with giant denominators are very small). For λ = ∞, the equation of Saha and Sinha subsequently takes the next type:

The primary a part of the equation is already just like Madhava’s system: you sum fractions with odd denominators. The final a part of the sum (–n)n – 1, nevertheless, is much less acquainted. The subscript n – 1 is the so-called Pochhammer image. Typically, the expression (a)n corresponds to the product a x(a + 1) x (a + 2) x … x (a + n – 1). For instance, (5)3 = 5 x 6 x 7 = 210. And the Pochhammer image within the above system subsequently ends in: (–n)n – 1 = (–n) x (–n + 1) x (–n + 2) x … x (–n + n – 3) x (–n + n – 2).
A Few Steps to Madhava’s System
All of those components look sophisticated at first, however they are often simplified rapidly. First, subtract –1 from every issue. The check in entrance of the large product is subsequently –1 if n is odd and +1 if n is even, so that you get (–n)n – 1 = (–1)n x n x (n – 1) x (n – 2) x … x (n – n + 3) x (n – n + 2). The final elements may be simplified additional: (–n)n – 1 = (–1)n x n x (n – 1) x (n – 2) x … x 3 x 2 x 1.
This elongated expression is definitely (–n)n – 1 = (–1)nx n, ensuing within the following:

This corresponds to Madhava’s system. The equation discovered by Saha and Sinha subsequently additionally comprises the collection found by Madhava.
As the 2 string theorists report, nevertheless, pi may be calculated a lot sooner for smaller values of λ. Whereas Madhava’s end result requires 100 phrases to get inside 0.01 of pi, Saha and Sinha’s system for λ = 3 solely requires the primary 4 summands. “While [Madhava’s] series takes 5 billion terms to converge to 10 decimal places, the new representation with λ between 10 [and] 100 takes 30 terms,” the authors write of their paper. Saha and Sinha didn’t discover essentially the most environment friendly technique for calculating pi, although. Different collection have been recognized for a number of a long time that present an astonishingly correct worth rather more rapidly. What is really shocking on this case is that the physicists got here up with a brand new pi system when their paper aimed to explain the interplay of strings. They developed a technique to point the chance with which two closed strings would work together with one another—one thing many string theorists have been searching for for many years with out success.
When Saha and Sinha took a better have a look at the ensuing equations, they realized that they might categorical the quantity pi on this manner, in addition to the zeta perform, which is the center of the Riemann conjecture, one of many best unsolved mysteries in arithmetic. Given the string theorists’ pursuits, their formulation for pi and the zeta perform solely adorn the final paragraph of their paper. “Our motivation, of course, was not to find a formula for pi,” Sinha stated in a YouTube video from Numberphile. “Pi was just a by-product.”
This text initially appeared in Spektrum der Wissenschaft and was reproduced with permission.

